An annual update on preparation for UK University Entrance Examinations for Mathematics; note that these resources provide challenging questions for any students anywhere studying Mathematics beyond age 16. (Teachers, see this page which includes a teacher webinar)
From Nrich, Prepare for University.
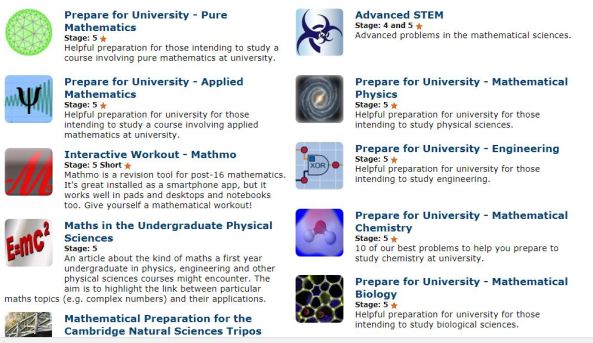

To further challenge yourself, MAT, STEP and AEA questions provide an excellent source of questions. Dr Jamie Frost has created such a useful resource with his STEP, MAT and AEA questions all aligned to new A Level chapters. This document is 156 pages of categorised questions (brief answers are given). Also available is a pdf file of just the STEP questions.
For mark schemes see:
- MAT (Maths Admissions Test), scroll down the page for past papers, solutions and additionally video solutions. For superb resources for these questions see these Underground Mathematics Review Questions where you will find not only the questions but suggestions and complete solutions.
- STEP mark schemes can also be found on the Cambridge Assessment Preparing for STEP page. Note that STEP 1 will no longer exist from Summer 2021.
- The AEA questions are legacy questions, extended solutions are currently available for the papers which have been used from the University of Warwick. Legacy papers and mark schemes are available on Colmanweb.
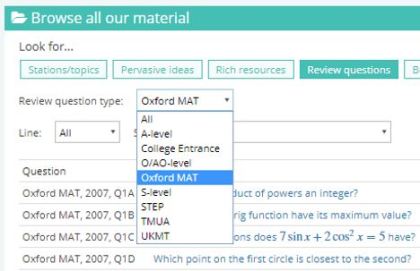
The Underground Mathematics Review Questions include Oxford Mathematics Admissions Test questions and full solutions.
TMUA is a newer admissions test only one question is available on the Underground Maths site, however, there is much overlap between the specifications for the TMUA and other tests such as the Oxford MAT, so these questions should provide useful resources for students taking this examination. Interestingly, Durham University states that “Those students already registered for MAT may substitute those results in place of our own test, if they do not wish to take both.”
Warwick University advise taking one of MAT, TMUA or STEP.

TUMA papers and mark schemes are available from Cambridge Assessment and I would highly recommend the presentation introducing the test, from Julian Gilbey. As suggested – try the questions first (pdf file) before watching the presentation.
Talking to Julian Gilbey, he recommends for the TMUA, the importance of working through the Extended specification notes on the website, to learn about the logic side. (See Test Specifications for the specification and enhanced specification with its notes on logic and proof.) He also stresses that the more Maths you can do, the more you work on stretching problems and think hard about maths the better you will get at maths. Examples he mentions for resources are any questions on the Underground Maths website, (not just the review questions already mentioned here), UKMT and olympiad problems, STEP problems.
“And essentially your ability to ‘think mathematically’ and to solve mathematical problems is all that these tests are testing”
For further resources try UKMT Senior Maths Challenge Questions.

…and also see MadAsMaths with its many papers and solutions increasing in difficulty.
You can download a free pdf copy of Stephen Siklos’ Advanced Problems in Mathematics. Whilst written to support students taking STEP examination papers, Advanced Problems in Mathematics is excellent preparation for any undergraduate Mathematics course.
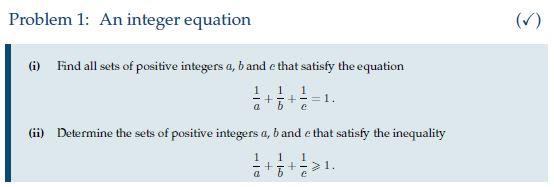

Following each question, you will find a discussion and a full solution. The clear Contents page lists all 75 problems.
STEP (Sixth Term Examination Paper) Mathematics is a well-established mathematics examination designed to test candidates on questions that are similar in style to undergraduate mathematics.
………..
You will find free STEP soluions on MEI’s site. The STEP question papers are all available on the Cambridge Assessment website; note the STEP resources include a searchable database. The AEA qualification from Pearson is based on the A Level specification and designed for the top 10% of students to help differentiate between the most able candidates. The Sample Assessment Materials provides a valuable source of challenging questions (with mark schemes) for A Level mathematicians.
STEP papers here. Underground Mathematics has STEP questions within their Review Questions. Each question comes with a fully worked solution.

See also, from Cambridge University, their STEP Support Programme. From the home page, access the resources, you will see STEP Support Programme Foundation modules, STEP 2 modules and STEP 3 modules.